Introduction
The conductivity σ is a characteristic of a liquid only if it is measured at thermodynamic equilibrium.
To fulfil this requirement high electric stress and/or prolonged voltage application is to be avoided, this is not the case in IEC 60247 for the d.c. resistivity measurement (electric stress up to 250 Vmm(-1), conventional arbitrary time of electrification 1 min).
There is a simple relationship between the dielectric dissipation factor tan δ, the conductivity σ and the permittivity ε of the liquid with no (or negligible) dipolar losses, which is the case of most liquids for electrotechnical applications:
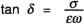
where ω = 2πf and f is the frequency of the voltage.
Therefore, the measurement of either tan δ or σ gives the same information on the conduction properties of the liquid. In fact, very often in practice, there are large discrepancies between the resistivity calculated from the measurement of tan δ with conventional apparatus and the d.c. resistivity measured following the recommendation of IEC 60247.
New devices for the measurement of the conductivity B at thermodynamic equilibrium are currently available. They are able to measure easily and with precision very low values of σ. The capabilities of this new equipment allow measurements of σ of unused insulating liquids even at room temperature.
1 Scope
This International Standard describes a method for the simultaneous measurement of conductance G and capacitance C enabling the calculation of the dielectric dissipation factor tan δ of insulating liquids. The proposed method applies both to unused insulating liquids and insulating liquids in service in transformers and in other electrical equipment.
The standard is no substitute for IEC 60247; rather it complements it insofar as it is particularly suited to highly insulating liquids and it recommends a method of measurement for these liquids. This method allows values of the dielectric dissipation factor as low as 10(-6) at power frequency to be determined with certainty. Moreover, the range of measurements of tan δ lies between 10(-6) and 1 and can be extended up to 200 in particular conditions.
2 Normative references
The following normative documents contain provisions which, through reference in this text, constitute provisions of this International Standard. At the time of publication, the editions indicated were valid. All normative documents are subject to revision, and parties to agreements based on this International Standard are encouraged to investigate the possibility of applying the most recent editions of the normative documents indicated below. Members of IEC and ISO maintain registers of currently valid International Standards.
IEC 60247:1978, Measurement of relative permittivity, dielectric dissipation factor and d.c. resistivity of insulating liquids.
IEC 60475:1974, Method of sampling liquid dielectrics.
ISO 5725-1:1994, Accuracy (trueness and precision) of measurement methods and results - Part 1: General principles and definitions.
ISO 5725-2:1994, Accuracy (trueness and precision) of measurement methods and results - Part 2: Basic method for the determination of repeatability and reproducibility of a standard measurement method.
ISO 5725-3:1994, Accuracy (trueness and precision) of measurement methods and results - Part 3: Intermediate measures of the precision of a standard measurement method.
ISO 5725-4:1994, Accuracy (trueness and precision) of measurement methods and results - Part 4: Basic methods for the determination of the trueness of a standard measurement method.
3 Definitions
For the purpose of this International Standard, the following definitions apply
3.1 conductivity (σ)
quantity related to the electric field strength E and to the conduction current density j by
j = σE
3.2 resistivity (ρ)
reciprocal of the conductivity σ, given by
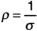
3.3 resistance (R)
the resistance of the liquid-filled test cell is the ratio of the voltage V applied to the cell to the direct or in-phase current IR, and is given by
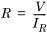
in the simplest case of plane parallel electrodes of area A and with a gap distance L,
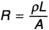
3.4 conductance (G)
reciprocal of the resistance, given by
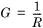
3.5 capacitance (C)
the capacitance of the liquid-filled test cell is the ratio of the charge Q of the electrodes to the voltage V applied to the test cell. For a plane capacitor,
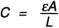
where ε is the permittivity of the liquid.
3.6 dielectric dissipation factor (dielectric loss tangent tan δ)
for a material subjected to a sinusoidal voltage, tan δ is the ratio of the value of the absorbed active power to the value of the reactive power. In the simple case of a capacitance C shunted by a resistance R,
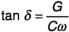
where ω = 2πf and f is the frequency of the voltage. details about the factors influencing the conduction of liquids can be found in Annex C.



