By John S. Evans, B.Sc.
Friction is a ubiquitous part of our daily lives. Walking, writing, opening doors and drawers and driving to work all involve energy being expended in order to overcome friction. Although friction is generally thought of as a negative mechanical characteristic (approximately 20% of a car's energy is spent in overcoming friction) it must be remembered that without it we would not be able to walk and the brakes on our cars would not work.
Friction is usually seen as a mechanical problem and the force necessary to overcome it has long interested engineers. When man invented the wheel it enabled him to move weights around with far less expenditure of energy than if they were dragged from A to B. What he had in fact done was to replace sliding friction with rolling friction which has a much lower value.
This bulletin looks at the role of oil in reducing the harmful forces of friction.
FRICTION
An understanding of friction will be helpful as a starting point. Friction can be defined as the resistance encountered when one body moves relative to another body with which it is in contact.
The laws and coefficient of friction
The basic laws of friction were 'sensed' by Leonardo da Vinci but were not studied scientifically until the 18th century by Coulomb and Amontons, which led to the definition of three laws:
The first law states that the force of friction that exists between two surfaces is directly proportional to the perpendicular pressure between them. In order to slide a metal cube of mass M across a table, a tangential force, F, must be applied. If M is doubled then F must also be doubled to get M to move.
The second law states that the force of friction is independent of the surface area of M in contact with the surface over which it is being made to slide. If M were to have an oblong cross section, it would not matter whether the larger or smaller surface area was placed on the table; the force of friction, or the force required to move both blocks, would remain the same. Diagram 1 illustrates this point.
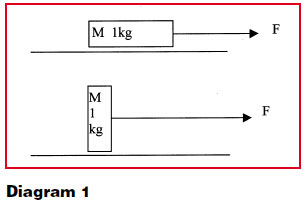
The third law says that the force of friction is dependent on the nature and state of the surfaces. Smooth surfaces generate less friction than rough surfaces.
The first law can be restated as the ratio between F and M for a particular system is constant and F/M is known as the coefficient of friction. This coefficient can be easily measured by using gravity to make a metal block slide across a surface. Although the mass can be weighed and the force to get it to move easily determined, inertia causes problems.
Newton's first law of motion can be interpreted as saying that bodies at rest tend to stay that way unless acted upon. This means that the initial energy required to get the metal block to start moving (overcome static friction) is higher than the energy required to keep it moving (overcome dynamic friction). Once the initial energy has been expended to overcome the static friction, the coefficient of friction settles down to a constant value equal to the dynamic friction. Thus frictional drag is lower once a body is in motion.



