6. Applying Precision Data to Test Methods
6.1 This section describes procedures in which the precision limits of test methods may be used to indicate when results obtained by two laboratories differ significantly. This section may also be used for rejection of results of replicate tests by an operator.
6.2 Significance of Repeatability (r):
6.2.1 Acceptance of Results - When only two results are obtained under conditions of repeatability and the difference is equal to or less than the repeatability of the method, the operator may report the average of the two results as being applicable to the sample tested.
6.2.2 Rejection of Results - When two results are obtained that differ by more than the repeatability of the method, both should be rejected. Obtain two additional results immediately under conditions of repeatability. If the difference between these two results is equal to or less than the repeatability of the method, the operator should report the average of the two as being applicable to the sample tested. If, however, the difference so obtained again exceeds the repeatability, reject the results and investigate the application of the method.
6.3 Significance of Reproducibility (R):
6.3.1 Acceptance of Results - When two results are obtained in different laboratories (Note 1) and the difference is equal to or less than the reproducibility of the method, both results should be considered acceptable. The value assigned to the sample should be the average of the two results.
NOTE 1 - When a comparison for reproducibility is made between results from two laboratories, it is assumed that single results from each will be compared. If each of the laboratories has produced more than a single result, see 6.4.
6.3.2 Rejection of Results - When the results from two laboratories differ by more than the reproducibility of the method, reject both results and each laboratory should repeat the test on the retained sample. If the difference is now equal to or less than the reproducibility, both results should be considered acceptable and their average reported. If, however, the difference between these results is still greater than the reproducibility, reject the results and investigate the application of the method at each laboratory.
6.4 Multiple Testing - If the number of results obtained in either one or both laboratories is more than one, then the allowable difference between the averages from the two laboratories is given as follows:
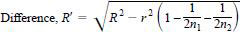
where:
R = reproducibility of the method,
r = repeatability of the method,
n1 = number of results of the first laboratory, and
n2 = number of results of the second laboratory.
6.5 Referee Laboratory - In the event a third or referee laboratory is invited to make the test using a portion of one of the samples described in 6.3.2, multiply the reproducibility, R, by 1.2 (to convert a range for two to a range for three) and compare this value with the difference between the two extreme results for acceptance. If acceptance is indicated, the assigned test value (ATV) for the sample should be the average of the three results.
7. Applying Precision Data to Specifications
7.1 Specifications - A specification fixes a limit to the true value of a given property. In practice, however, this true value can never be established exactly. The property is measured in the laboratory by applying a standard test method, the results of which may show some scattering as defined by the repeatability and reproducibility limits. There is always, therefore, some uncertainty as to the true value of the tested property.
7.2 Although the true value is never known exactly, the probability of obtaining any specific test result, relative to the true value, can be calculated if the probability distribution function for the test method is known (for example, the normal distribution curve with its associated reproducibility).
7.2.1 Some specifications, because of the product characteristic or the end use of the product, or both, require that the receiver have a high degree of assurance that the product actually meets or exceeds the quality level indicated by the specification value. For the purpose of this practice, such specifications are called critical specifications.
7.2.2 Specifications that require assurance only that the product quality is not substantially poorer than is indicated by the specification level are called noncritical specifications for the purposes of this practice.
7.3 Specification Conformance Guidelines:
7.3.1 Whenever a product is tested for conformity to a specification, a decision must ultimately be made as to the acceptance or rejection of the product.
7.3.2 The numerical value that divides the regions of acceptable and unacceptable product test values is the acceptance limit (AL). The AL may or may not coincide with the specification value (S) used to define a product quality or grade.
7.3.3 The AL value, which must be agreed upon by the supplier and receiver, is that level of quality such that, if the true value is exactly AL, they are willing to take a 50 % chance of either accepting or rejecting the product as tested.
7.3.4 In the absence of an agreement to the contrary, the specification will be considered a noncritical specification for which there is 95 % assurance that the product will be accepted if the true quality is the specification value. Thus, the AL will be set by using a confidence level P = 0.95 as shown in 7.3.6.
7.3.5 The probability of accepting a product (deciding that product quality is acceptable) when the true value equals the specification value is shown in Table 1 and Fig. 1 as a function of D = (AL - S)/0.255R, a direct measure of the difference between AL and S. This relationship is based (1) on the assumption of normally (Gaussian) distributed testing errors, which is adequate for most test procedures, and (2) on using an assigned test value (ATV) for making the specification conformance decision that is the average of precision-acceptable results from two laboratories.
7.3.6 Instead of deciding directly on an AL, the receiver may select a given probability P of accepting the product when the true value equals the specification value S. From the relationship given, read a value D corresponding to the ordinate value P. The proper AL is then given by
AL = S + 0.255 x R x D
For N, other than two different laboratory results, the 0.255 factor should be multiplied by √2/N .
7.3.6.1 For specifications having both minimum and maximum limits, the procedure in 7.3.6 must be applied twice to give both upper and lower ALs. There must be some allowable region remaining between the lower and upper ALs.
7.3.7 When only a single test result is or will be available, the relationships given should be used with N = 1 (7.3.6). Obviously, no check on reproducibility precision can be made with a single test result, and the single value becomes the ATV for the sample.
7.3.8 The AL for critical specifications is thus set so that if the true value is less than or equal to S, there is only a small probability (defined by selection of P) of accepting the product.
7.3.9 For noncritical specifications, the AL is set so that if the true value is equal to or greater than S, there is a high probability (defined by selection of P) of accepting the product.
7.3.10 The relationships between the ALs for critical and noncritical specifications are shown in Fig. 2 for a minimum specification.



