11. Calculations
11.1 Aromatic Fraction - Read peak heights from the record mass spectrum corresponding to m/e + ratios of 67 to 69, 71, 81 to 83, 85, 91, 92, 96, 97, 103 to 106, 115 to 120, 128 to 134, 141 to 148, 151 to 162, 165 to 198, 203 to 212, 217 to 226, 231 to 240, 245, 246, 247 to 252.
Find:
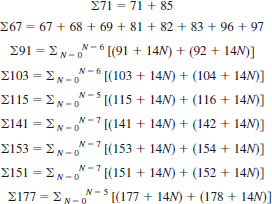
11.2 Calculate the mole fraction at each carbon number of the alkylbenzenes for n = 10 to n = 18 as follows:
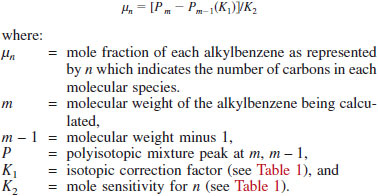
NOTE 5 - This step of calculation assumes no mass spectral pattern contributions from other hydrocarbon types to the parent and parent-1 peaks of the alkylbenzenes. Selection of the lowest carbon number 10 is based upon the fact that C9 alkylbenzenes boil below 204°C (400°F) and their concentration can be considered negligible.
11.3 Find the average carbon number of the alkylbenzenes, A, in the aromatic fraction as follows:
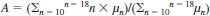
11.4 Calculate the mole fraction at each carbon number of the naphthalenes for n = 11 to n = 18 as follows:
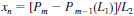
where:
xn = mole fraction of each naphthalene as represented by n which indicates the number of carbons in each molecular species,
m = molecular weight of the naphthalenes being calculated,
m - 1 = molecular weight minus 1,
P = polyisotopic mixture peak at m, m - 1,
L1 = isotopic correction factor (see Table 1), and
L2 = mole sensitivity for n (see Table 1).
NOTE 6 - This step of calculation assumes no mass spectral pattern contributions to the parent and parent-1 peaks of the naphthalenes. The concentration of naphthalene itself at a molecular weight of 128 shall be determined separately from the polyisotopic peak at m/e+ 128 in the matrix calculation. The average carbon number for the naphthalenes shall be calculated from carbon number 11 (molecular weight 142) to 18 (molecular weight 240).
11.5 Find the average carbon number of the naphthalenes, B, in the aromatic fraction as follows:
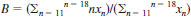
11.6 Selection of pattern and sensitivity data for matrix carbon number of the types present. The average carbon number of the paraffins and cycloparaffins (∑71 and ∑67, respectively) are related to the calculated average carbon of the alkylbenzenes (11.3), as shown in Table 2. Both ∑71 and ∑67 are included in the aromatic fraction matrix to check on possible overlap in the separation. The other types present, represented by ∑'s 103, 115, 153, and 151, are usually relatively low in concentration so that their parent ions are affected by other types present. The calculation of their average carbon number is not straight forward. Therefore, their average carbon numbers are estimated by inspection of the aromatic spectrum. Generally, their average carbon numbers may be taken to be equivalent to that of the naphthalenes, or to the closest whole number thereof, as calculated in 11.5. The average carbon number of tricyclic aromatics ∑177 has to be at least C14 and in full boiling range middle distillates C14 may be used to represent the ∑177 types carbon number. From the calculated and estimated average carbon numbers of the hydrocarbon types, a matrix for the aromatic fraction is set up using the calibration data given in Table 3. A sample matrix for the aromatic fraction is shown in Table 4. The matrix calculations consist in solving a set of simultaneous linear equations. The pattern coefficients are listed in Table 3. The constants are the ∑ values determined from the mass spectrum. Second approximation solutions are of sufficient accuracy. If many analyses are performed using the same type of a matrix, the matrix may be inverted for simpler, more rapid desk calculation. Matrices may also be programmed for automatic computer operations. The results of matrix calculations are converted to mass fractions by dividing by mass sensitivity. The mass fractions are normalized to the mass percent of the aromatic fraction, as determined by the separation procedure.
11.7 Saturate Fraction - Read peak at heights from the record of the mass spectrum corresponding to m/e+ ratios of 67 to 69, 71, 81 to 83, 85, 91, 92, 96, 97, 105, 106, 119, 120, 123, 124, 133, 134, 137, 138, 147 to 152, 161 to 166, 175 to 180, 191 to 194, 205 to 208, 219 to 222, 233 to 236, 247 to 250.
Find:
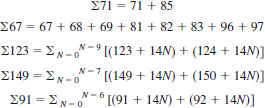
11.8 Selection of the pattern and sensitivity data for matrix calculation is dependent upon the average carbon number of the types present. The average carbon number of the paraffins and cycloparaffin types (∑'s 71, 69, 123, and 149), are related to the calculated average carbon number of the alkylbenzenes of the aromatic fraction (11.3), as shown in Table 2. The ∑91 is included in the saturate fraction as a check on the efficiency of the separation procedure. The pattern and sensitivity data for the ∑91 are based on the calculated or estimated average carbon number from the mass spectra of the aromatic fraction (see 11.3). From the determined average carbon numbers of the hydrocarbon types, a matrix for the saturate fraction is set up using the calibration data given in Table 3. A sample matrix for the saturate fraction is shown in Table 5. The matrix calculations of the saturate fraction consists in solving a set of simultaneous linear equations. The results of the matrix calculations (second approximation solutions are sufficient) are converted to mass fractions by dividing by mass sensitivity. The mass fractions are normalized to the mass percent of the saturate fraction as determined by the separation procedure.



